antiderivative A function F(x) is called an antiderivative of a function f(x) if F'(x) =; f(x) for all x in the domain of f. In words, this means that an antiderivative of f is a function which has f for its derivative.
chain rule The chain rule tells how to find the derivative of composite functions. In symbols, the chain rule says
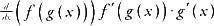
In words, the chain rule says the derivative of a composite function is the derivative of the outside function, done to the inside function, times the derivative of the inside function.
change of variables A term sometimes used for the technique of integration by substitution.
concave downward A function is concave downward on an interval if f"(x) is negative for every point on that interval.
concave upward A function is concave upward on an interval if f"(x) is positive for every point on that interval.
continuous A function f(x) is continuous at a point x =; c when f(c) exists, [img id:59930] exists, and [img id:59931]. In words, this means the curve could be drawn without lifting the pencil. To say that a function is continuous on some interval means that it is continuous at each point in that interval.
critical point A critical point of a function is a point (x, f(x)) with x in the domain of the function and either f'(x) =; 0 or f'(x) undefined. Critical points are among the candidates to be maximum or minimum values of a function.
cylindrical shell method A procedure for finding the volume of a solid of revolution by treating it as a collection of nested thin rings.
definite integral The definite integral of f(x) between x =; a and x =; b, denoted
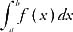
gives the signed area between f (x) and the x-axis from x =; a to x =; b, with area above the x-axis counting positive and area below the x-axis counting negative.
derivative The derivative of a function f (x) is a function that gives the slope of f (x) at each value of x. The derivative is most often denoted [img id:59928]. The mathematical definition of the derivative is
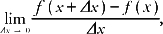
or in words the limit of the slopes of the secant lines through the point (x, f(x)) and a second point on the graph of f(x) as that second point approaches the first. The derivative can be interpreted as the slope of a line tangent to the function, the instantaneous velocity of the function, or the instantaneous rate of change of the function.
differentiable A function is said to be differentiable at a point when the function's derivative exists at that point. A function will fail to be differentiable at places where the function is not continuous or where the function has corners.
disk method A procedure for finding the volume of a solid of revolution by treating it as a collection of thin slices with circular cross sections.
Extreme Value Theorem A theorem stating that a function which is continuous on a closed interval [a, b] must have a maximum and a minimum value on [a, b].
First Derivative Test for Local Extrema A method used to determine whether a critical point of a function is a local maximum or local minimum. If a continuous function changes from increasing (first derivative positive) to decreasing (first derivative negative) at a point, then that point is a local maximum. If a function changes from decreasing (first derivative negative) to increasing (first derivative positive) at a point, then that point is a local minimum.
general antiderivative If F(x) is an antiderivative of a function f(x), then F(x) + C is called the general antiderivative of f(x).
general form The general form (sometimes also called standard form) for the equation of a line is ax + by =; c, where a and b are not both zero.
higher order derivatives The second derivative, third derivative, and so forth for some function.
implicit differentiation A procedure for finding the derivative of a function which has not been given explicitly in the form "f(x) =;".
indefinite integral The indefinite integral of f(x) is another term for the general antiderivative of f(x). The indefinite integral of f (x) is represented in symbols as
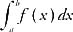
instantaneous rate of change One way of interpreting the derivative of a function is to understand it as the instantaneous rate of change of that function, the limit of the average rates of change between a fixed point and other points on the curve that get closer and closer to the fixed point.
instantaneous velocity One way of interpreting the derivative of a function s(t) is to understand it as the velocity at a given moment t of an object whose position is given by the function s(t).
integration by parts One of the most common techniques of integration, used to reduce complicated integrals into one of the basic integration forms.
intercept form The intercept form for the equation of a line is x/a + y/b =; 1, where the line has its x-intercept (the place where the line crosses the x-axis) at the point (a,0) and its y-intercept (the place where the line crosses the y-axis) at the point (0,b).
limit A function f(x) has the value L for its limit as x approaches c if as the value of x gets closer and closer to c, the value of f(x) gets closer and closer to L.
Mean Value Theorem If a function f(x) is continuous on a closed interval [a,b] and differentiable on the open interval (a,b), then there exists some c in the interval [a,b] for which
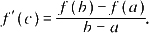
normal line The normal line to a curve at a point is the line perpendicular to the tangent line at that point.
point of inflection A point is called a point of inflection of a function if the function changes from concave upward to concave downward, or vice versa, at that point.
point-slope form The point-slope form for the equation of a line is y – y1 =; m(x – x1), where m stands for the slope of the line and (x1,y1) is a point on the line.
Riemann sum A Riemann sum is a sum of several terms, each of the form f(xi)Δx, each representing the area below a function f(x) on some interval if f(x) is positive or the negative of that area if f(x) is negative. The definite integral is mathematically defined to be the limit of such a Riemann sum as the number of terms approaches infinity.
Second Derivative Test for Local Extrema A method used to determine whether a critical point of a function is a local maximum or local minimum. If f'(x) =; 0 and the second derivative is positive at this point, then the point is a local minimum. If f'(x) =; 0 and the second derivative is negative at this point, then the point is a local maximum.
slope of the tangent line One way of interpreting the derivative of a function is to understand it as the slope of a line tangent to the function.
slope-intercept form The slope-intercept form for the equation of a line is y =; mx + b, where m stands for the slope of the line and the line has its y-intercept (the place where the line crosses the y-axis) at the point (0,b).
standard form The standard form (sometimes also called general form) for the equation of a line is ax + by =; c, where a and b are not both zero.
substitution Integration by substitution is one of the most common techniques of integration, used to reduce complicated integrals into one of the basic integration forms.
tangent line The tangent line to a function is a straight line that just touches the function at a particular point and has the same slope as the function at that point.
trigonometric substitution A technique of integration where a substitution involving a trigonometric function is used to integrate a function involving a radical.
washer method A procedure for finding the volume of a solid of revolution by treating it as a collection of thin slices with cross sections shaped like washers.